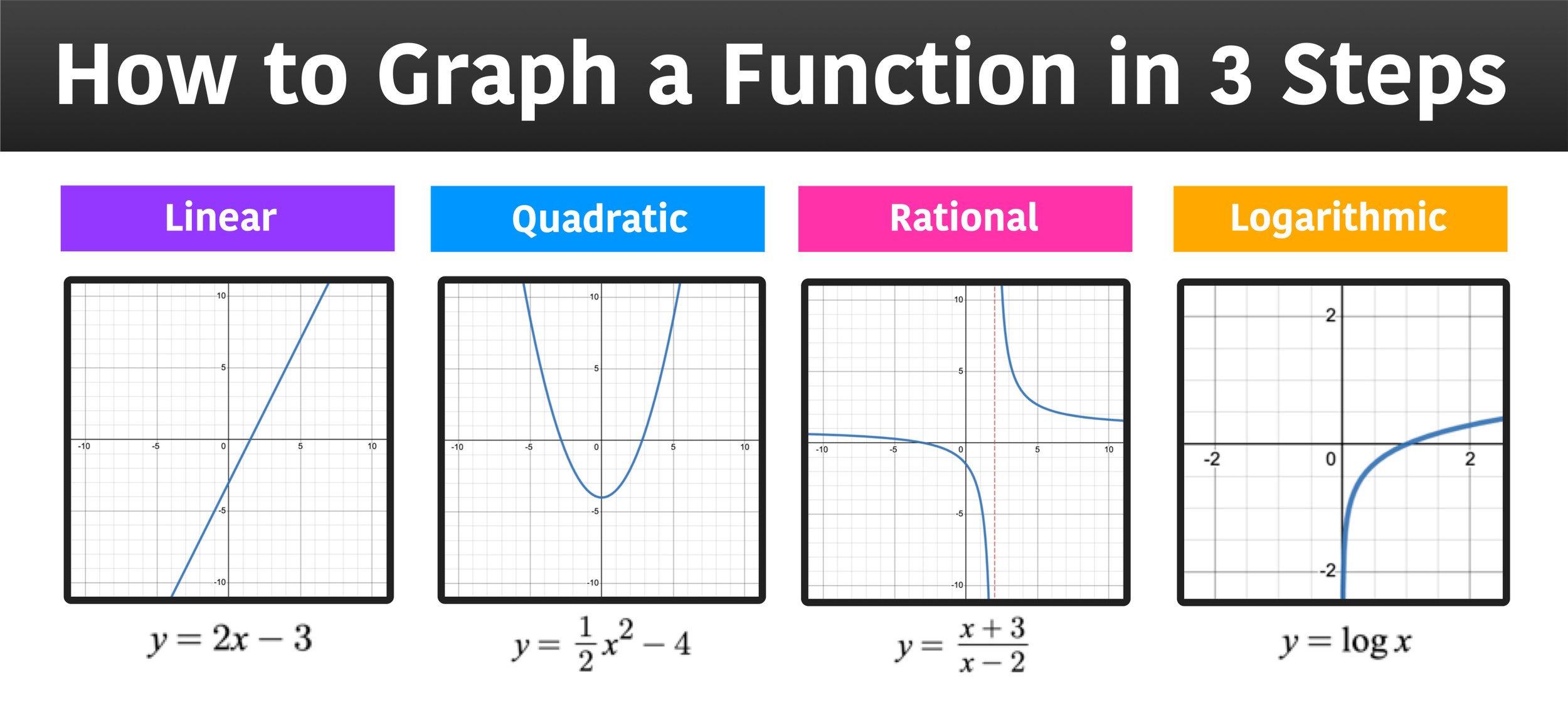
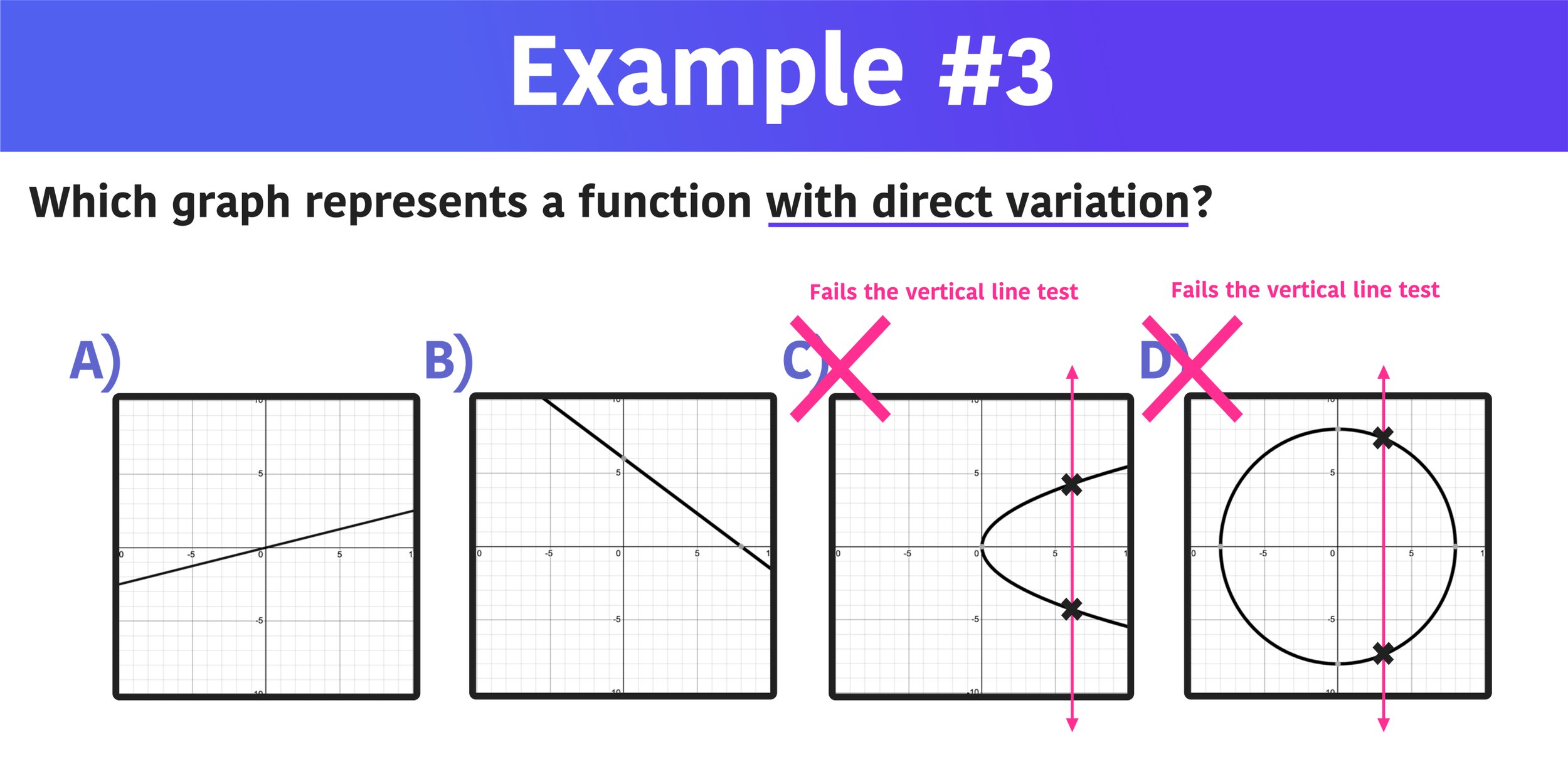
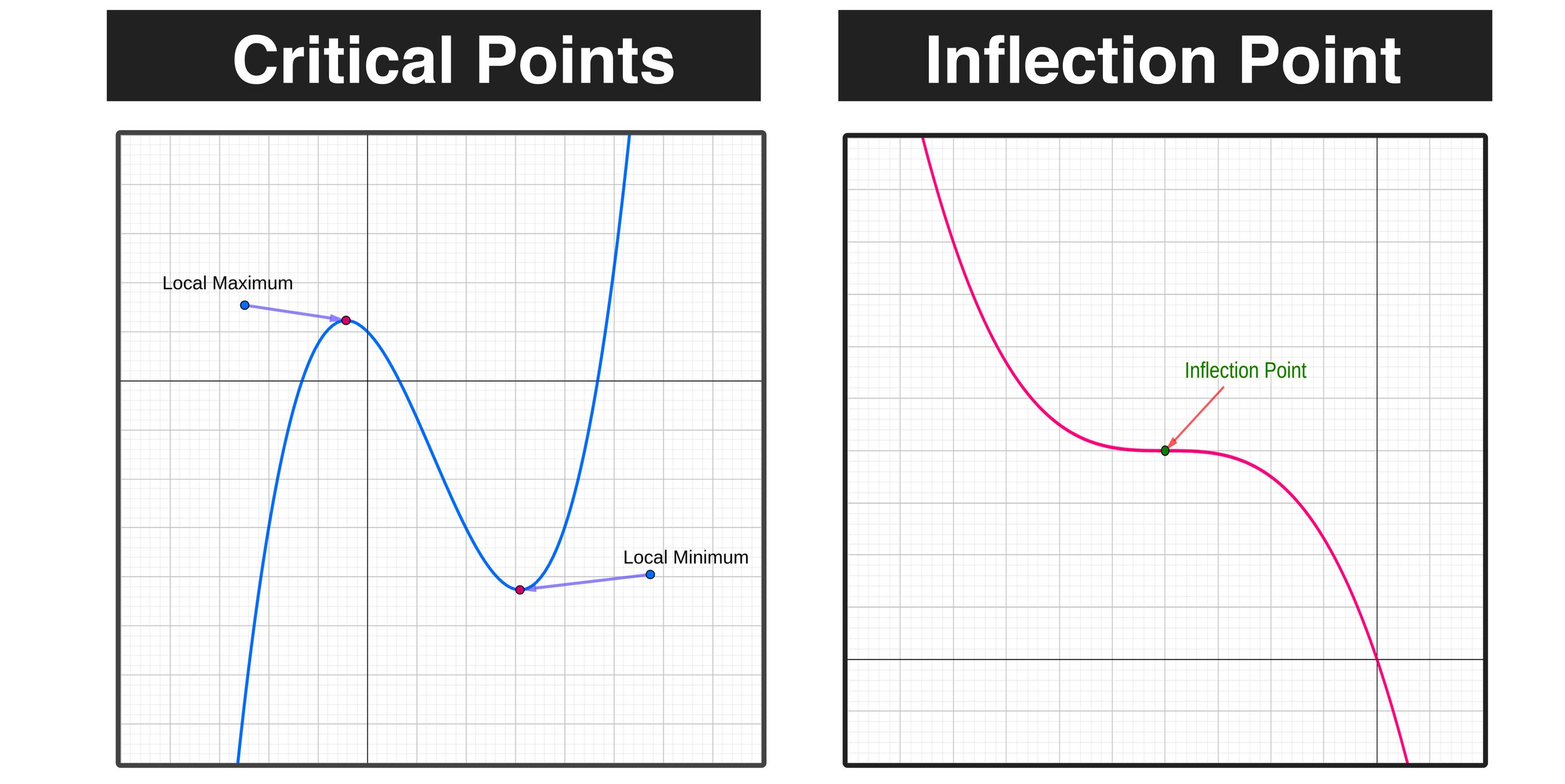
Here’s A Quick Way To Solve A Tips About How To Know If A Graph Is Smooth Position Time

Explore math with our beautiful, free online graphing calculator.

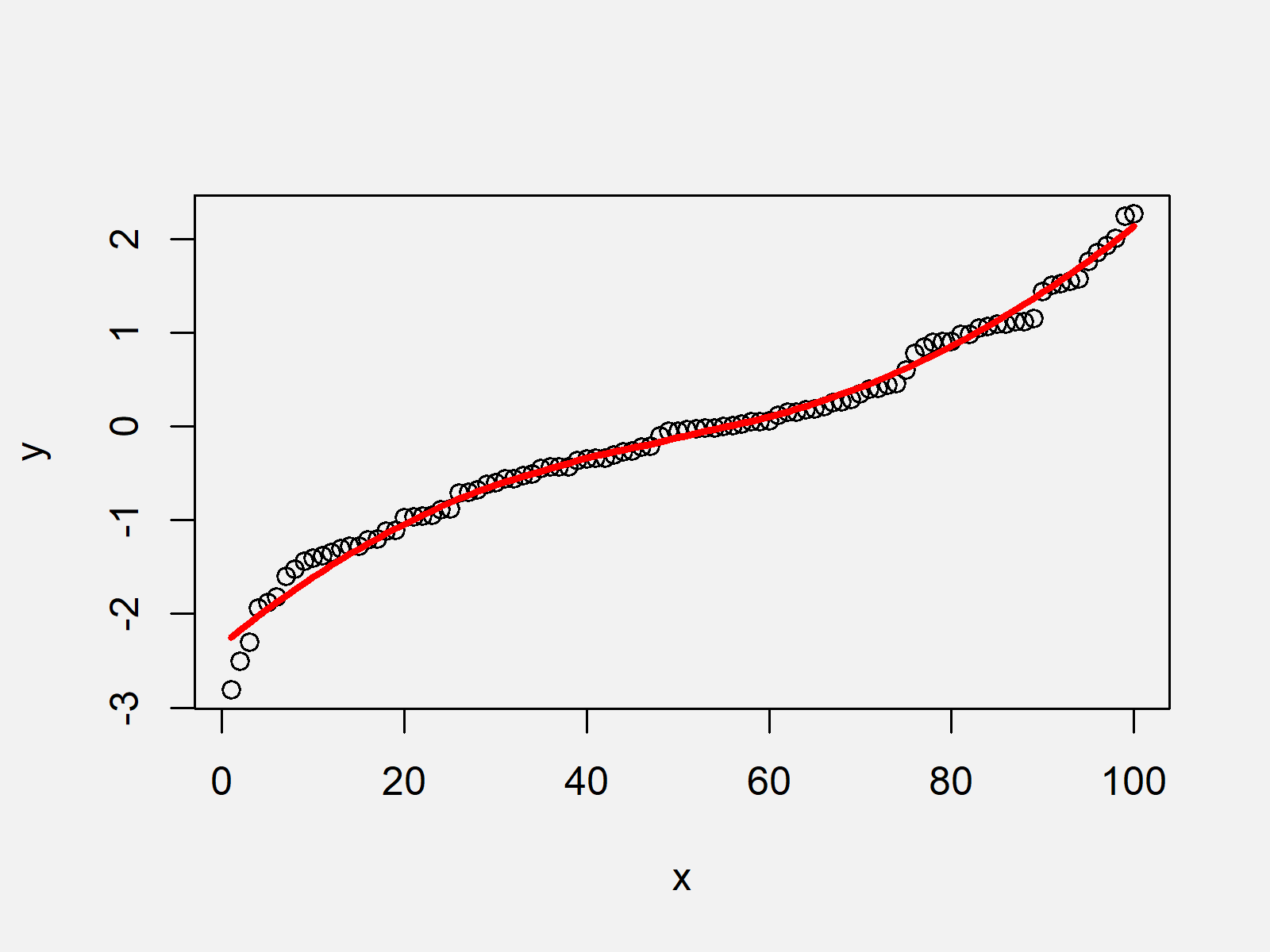
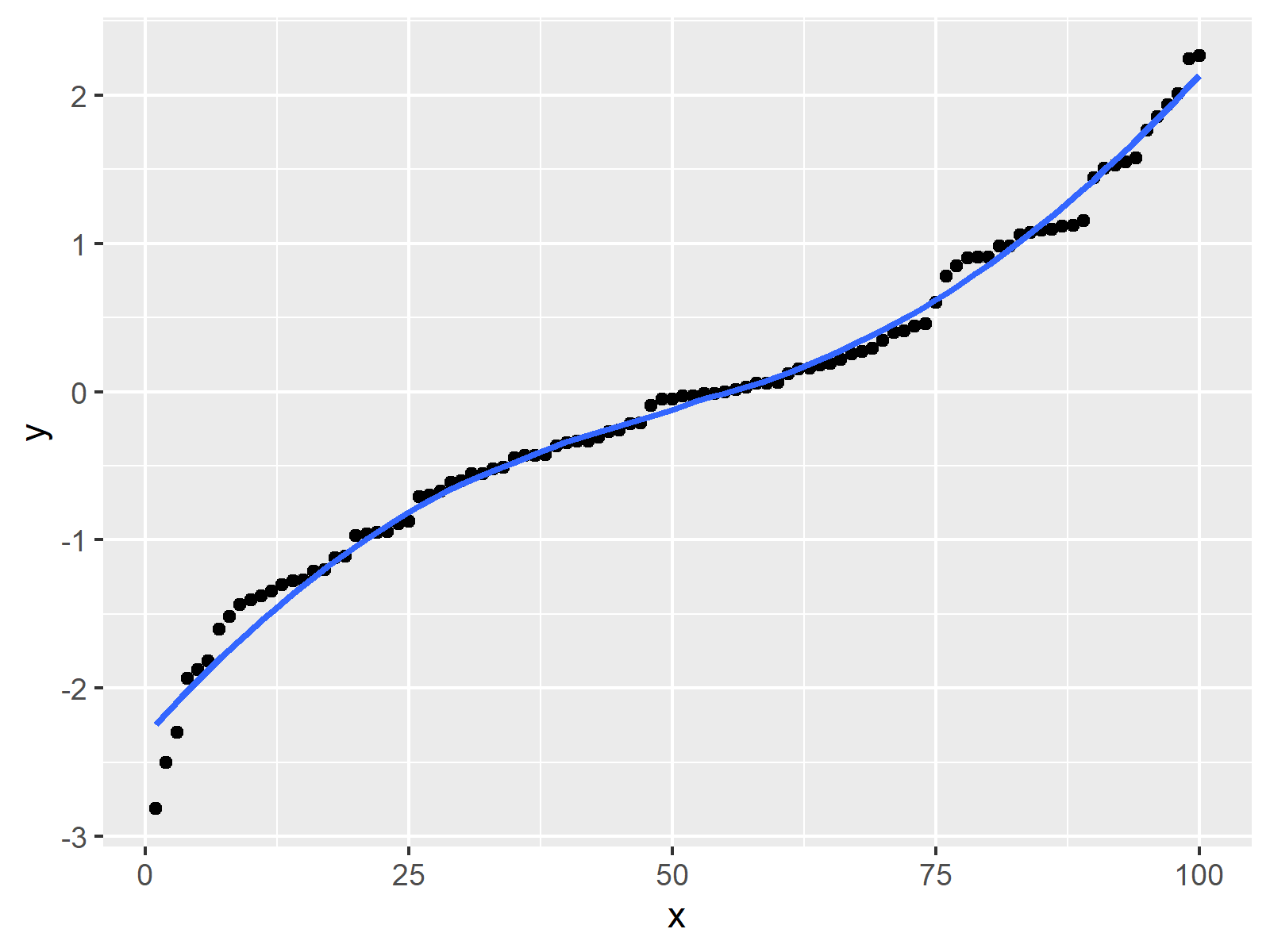
How to know if a graph is smooth. Let’s start with a graph showing the global temperature anomaly between 1880 and 2022 [2]. This video explains how to determine when a curve express by a vector valued function is smooth. Graph smoothing, also known as smoothing away or smoothing out, is the process of replacing edges e^'=v_iv_j and e^ ('')=v_jv_k incident at a vertex v_j of vertex.

The algorithm for identifying whether or not a parametric curve is smooth contains a first step of finding out when dx/dt and dy/dt is simultaneously 0. 1 , x3(6x2 − 15x + 10.) to save your graphs! For instance, take the function f(x, y) =y2.

A curve $\mathbf{r}(t)$ is considered to be smooth if its derivative, $\mathbf{r}'(t)$, is continuous and nonzero for all values of $t$. Let's dive into examples of functions and their graphs, focusing on finding points where the function isn't differentiable. You need to carefully choose the cutoff frequency for the right level of smoothing.

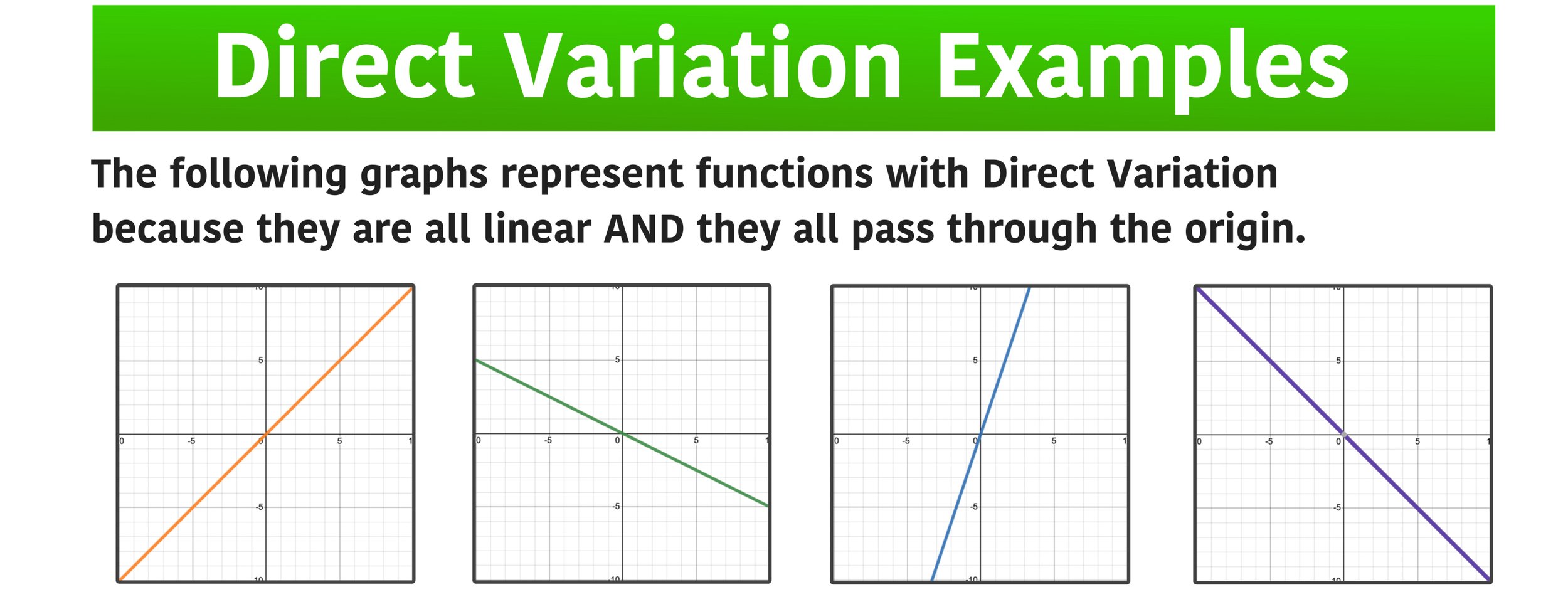
A smooth function must be differentiable, and the derivative must be continuous. Graph functions, plot points, visualize algebraic. It depends on the specific use case.

But often you'll find that what. Smooth functions have a unique defined first derivative (slope or gradient) at every point. A set $s$ is a smooth curve if (a) $s$ is.

If it's important to display the exact values, then the straight lines do a much better job. This is a very simple kind of filtering (box filtering in frequency domain), so. If ∇f(x, y) = 0 ∇ f ( x, y) = 0 at the point (x, y) ( x, y), then the theorem may fail to hold.

X = x < 0 : Graphically, a smooth function of a single variable can. Complex) projective plane if the system has no other real (resp.

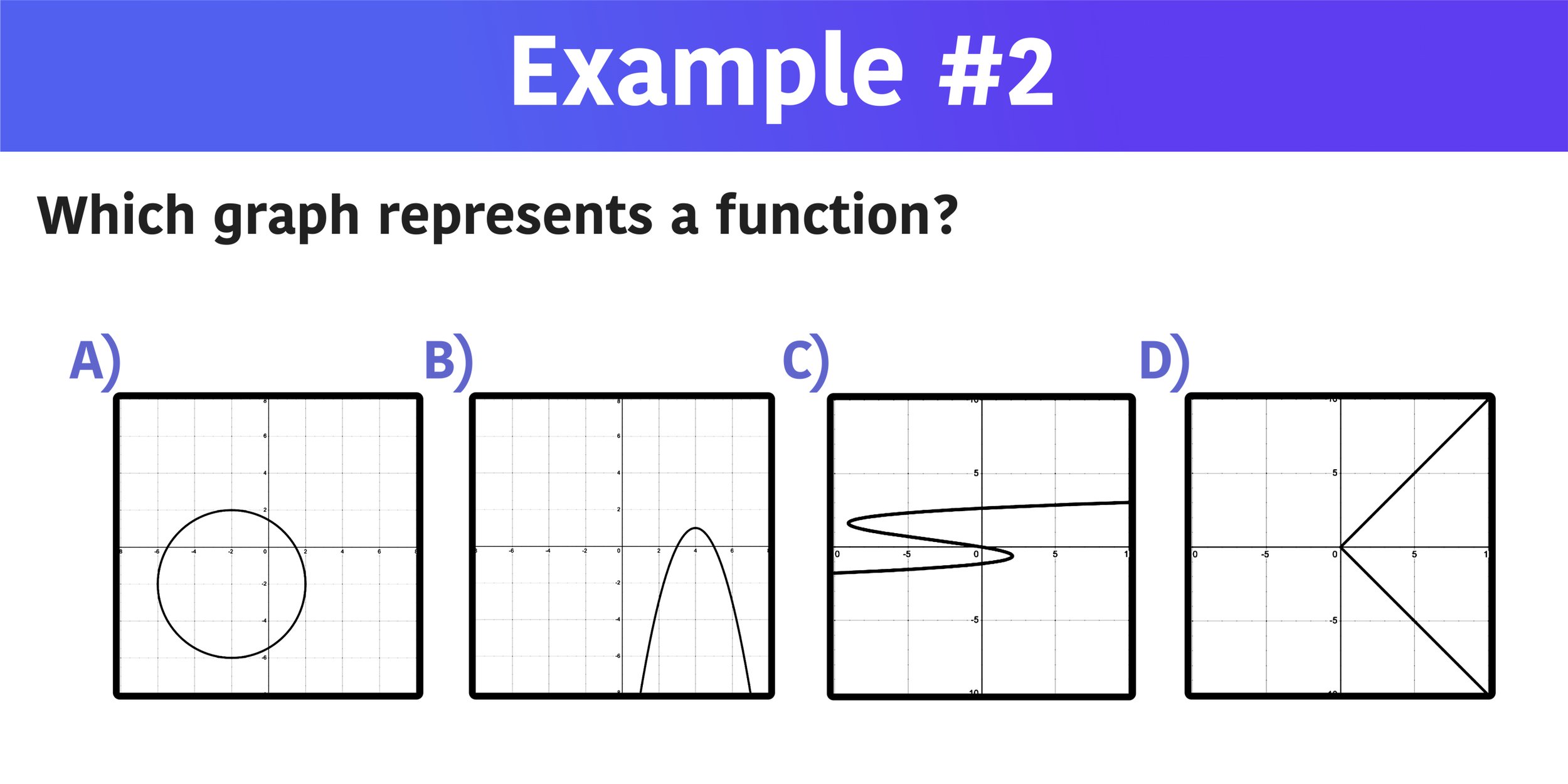
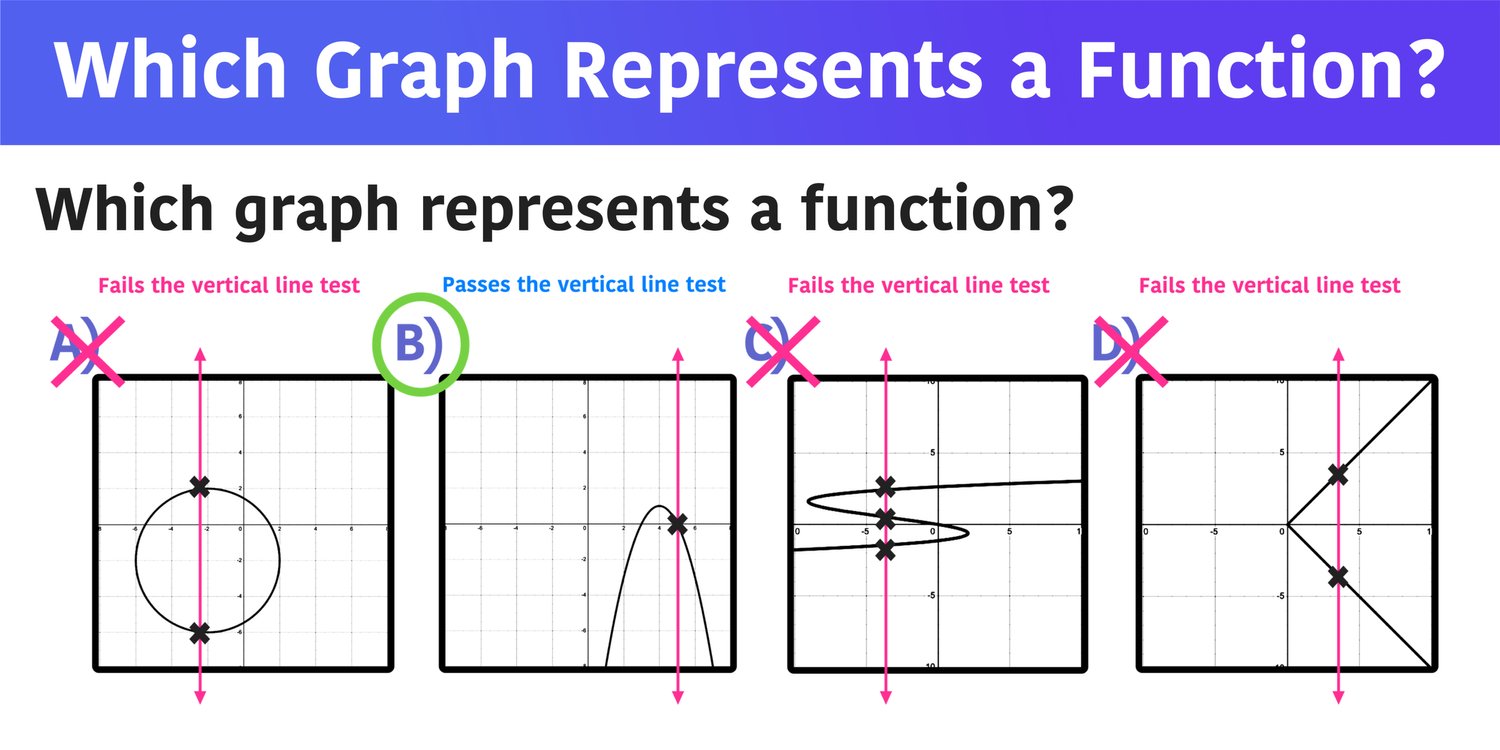
I have to determine whether the following curves are smooth or not and i'm having trouble with the following two functions: A graph for a function that's smooth without any holes, jumps, or asymptotes is called continuous. In orange is the measured data and in green is the same data.

The formal definition of a smooth curve is: Let's consider the first theorem. 0 , x > 1 :

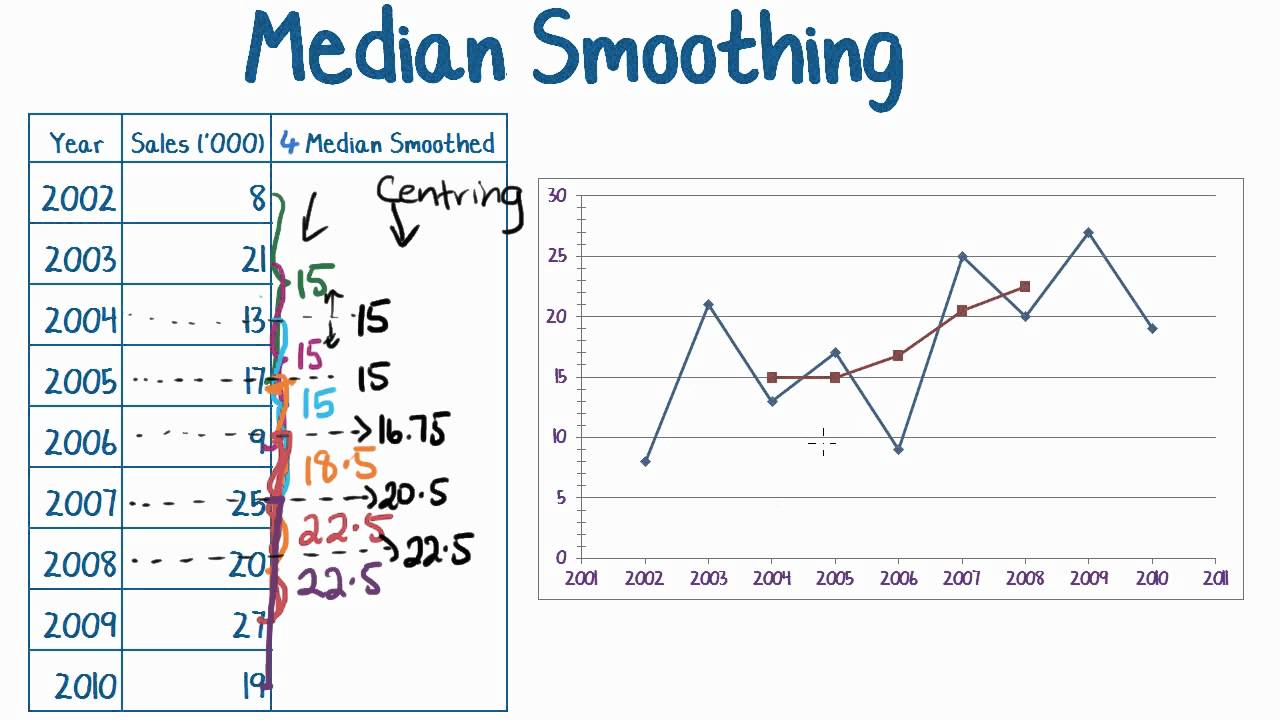
A clear definition of smoothing of a 1d signal from scipy cookbook shows you how it works. Consider the following curve in the plane, $(x(t),y(t))$, this curve is called smooth if the functions $x(t)$ and $y(t)$ are smooth, which simply means that for all $n$, the derivatives $\frac{d^nx}{dt^n}$ and $\frac{d^ny}{dt^n}$ exist. By examining various cases such as vertical tangents,.