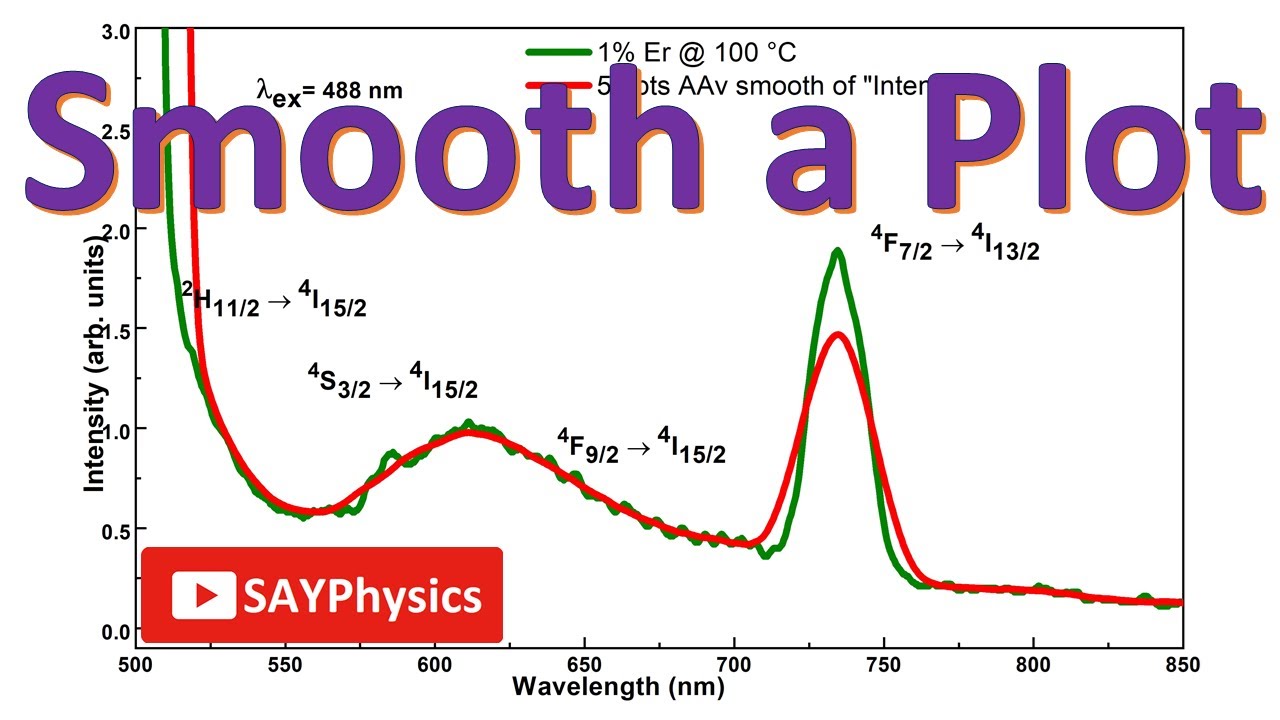
Build A Tips About How To Tell If A Graph Is Smooth Stacked Horizontal Bar

(b) every a ∈ s a ∈ s has a neighborhood n n such s s and n n is the graph of a c1 c 1 function f f. the question seems obvious if we.

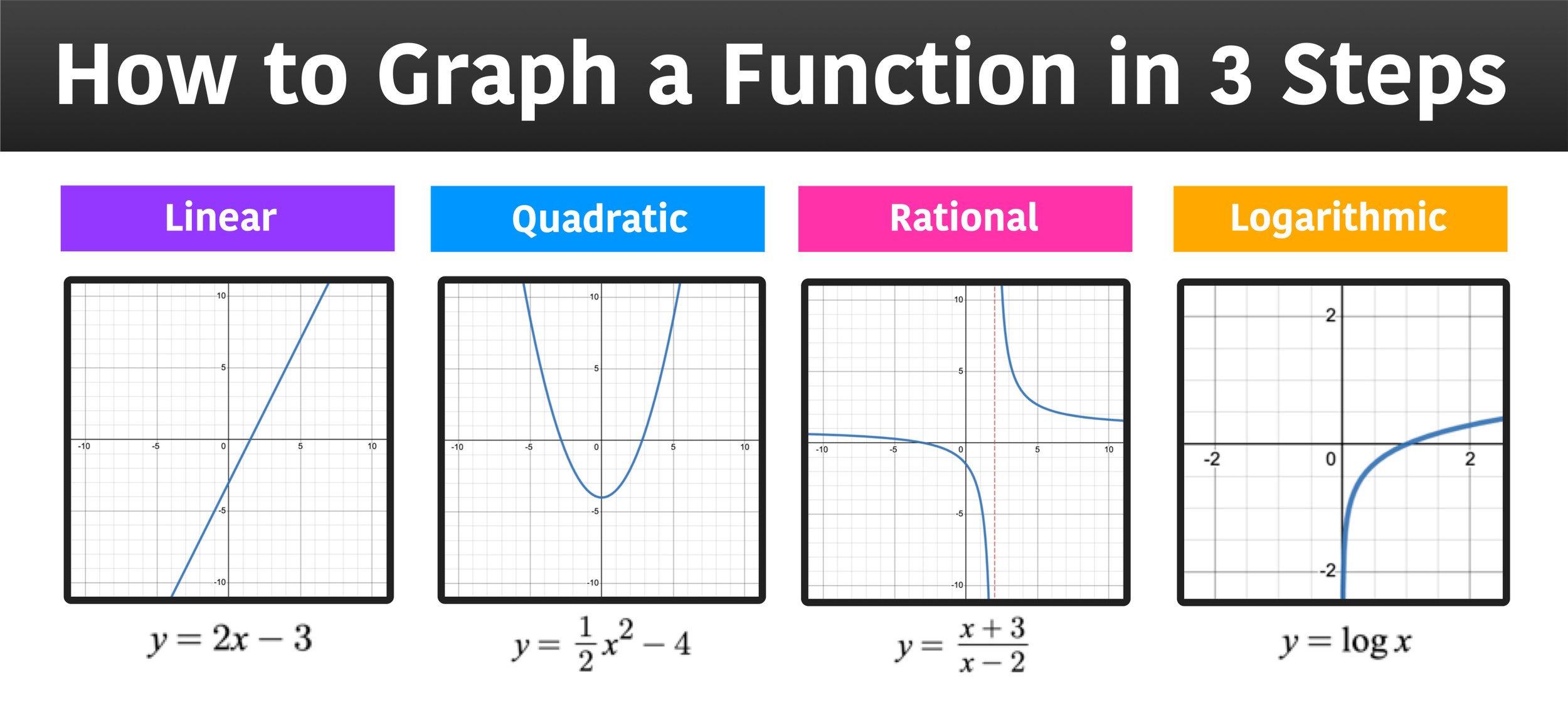
How to tell if a graph is smooth. A smooth curve is a curve which is a smooth function, where the word curve is interpreted in the analytic geometry context. A continuous function has no breaks in its graph: Smooth usually means no rough edges or corners.

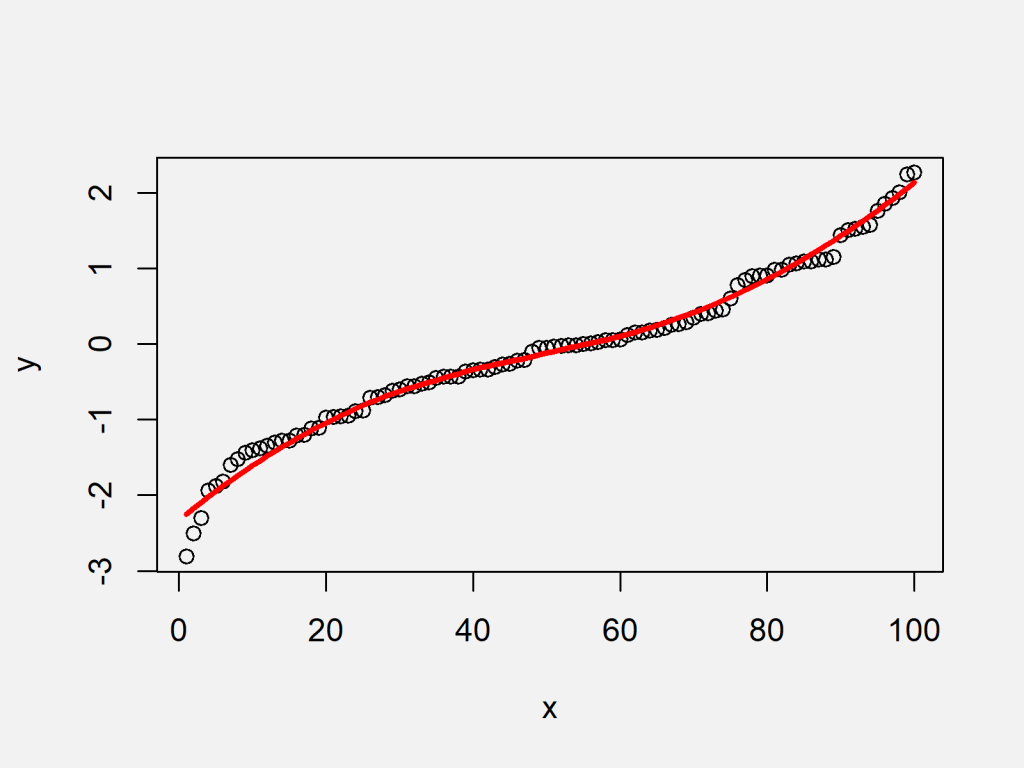
Smooth functions have a unique defined first derivative (slope or gradient) at every point. You choose the number of neighboring points to average and the 'order' of the smoothing polynomial. The turning points of a smooth graph must always occur at rounded curves.

You can argue that $g(x)$ is infinitely many times smooth. Graph smoothing, also known as smoothing away or smoothing out, is the process of replacing edges e^'=v_iv_j and e^ ('')=v_jv_k incident at a vertex v_j of vertex degree 2 by a single new edge e=v_iv_k and removing. A set s s is a smooth curve if.

In this video, i show that a curve described by a vector function is not smooth by showing there are values of t that make the derivative equal to zero. Graphically, a smooth function of a single variable can be plotted as a single continuous line with no abrupt bends or breaks. A curve $\mathbf{r}(t)$ is considered to be smooth if its derivative, $\mathbf{r}'(t)$, is continuous and nonzero for all values of $t$.

Take $f(x) = x|x|$ it is smooth, and now consider $g(x) = x^3$, this other function is also smooth. A smooth curve is a graph that has no sharp corners. Explore math with our beautiful, free online graphing calculator.

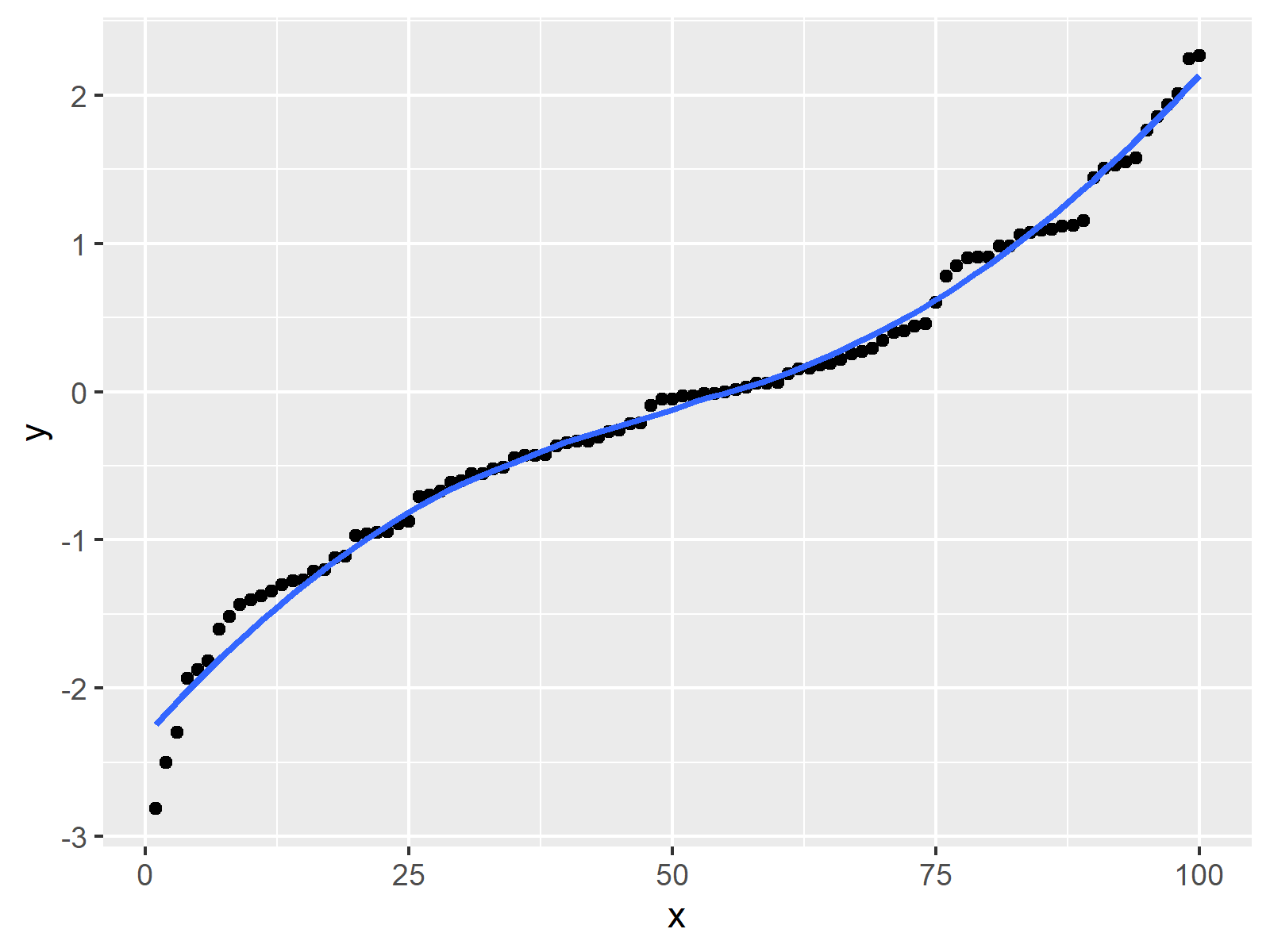
This method is based on the convolution of a scaled window with the signal. Let's examine the curves $\mathbf{r}_1(t) = \langle t,t\rangle$ and $\mathbf{r}_2(t) = \langle t^3,t^3\rangle$. A straight line suggests that the value between the two measurements increased linearly, while a curved line suggests otherwise.

For example, the following graph is smooth: You can't decide whether to use a straight line or a curved line between data points because you have no data to tell you what the shape of the line should be. This one is not smooth:

$f'(t) = (2t,2t)^{t}=$ 0 iff $t=0$. For this, one has to clarify whether the curve is considered in the real affine plane, the real projective plane, or the complex projective plane, the conditions getting stronger and stronger. This video explains how to determine when a curve express by a vector valued function is smooth.

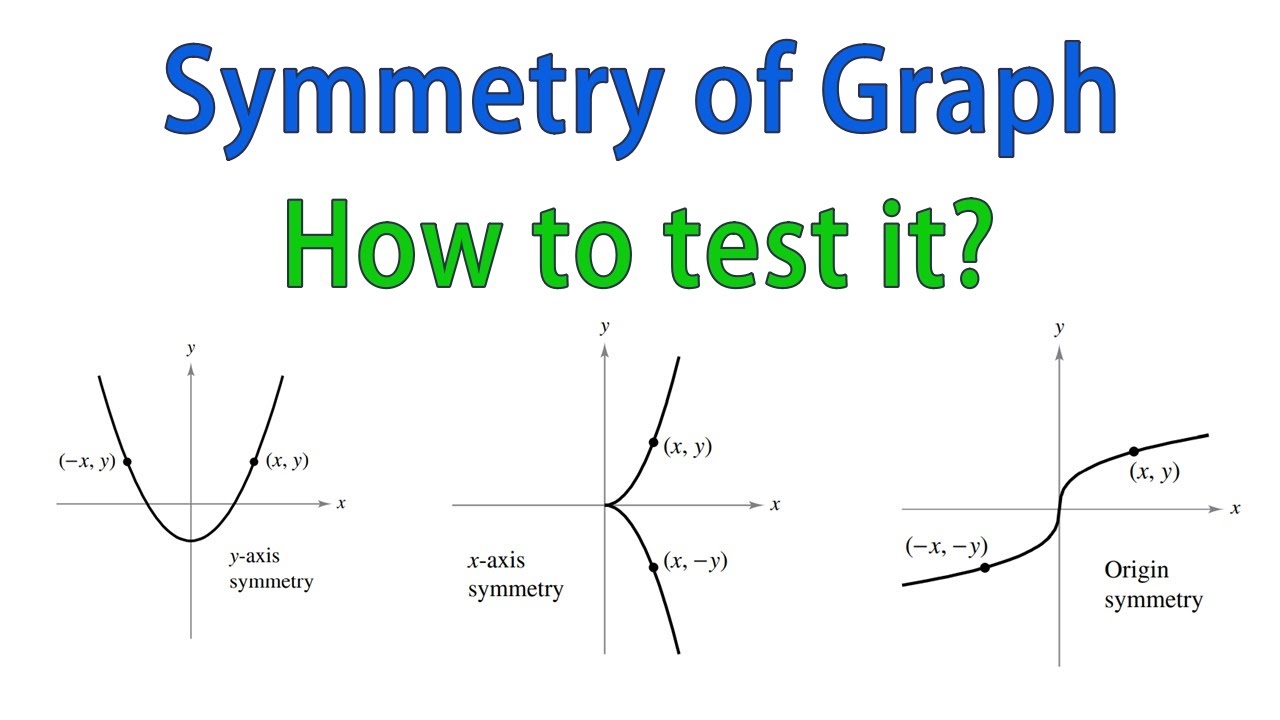
Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. A curve is said to be smooth if it has no singular points, in other words if it has a (unique) tangent at all points. Y0) on the graph, and zoom in.

Prism gives you two ways to adjust the smoothness of the curve. Smoothing is not a method of data analysis, but is purely a way to create a more attractive graph. Consider the following curve in the plane, $(x(t),y(t))$, this curve is called smooth if the functions $x(t)$ and $y(t)$ are smooth, which simply means that for all $n$, the derivatives $\frac{d^nx}{dt^n}$ and $\frac{d^ny}{dt^n}$ exist.