Brilliant Tips About How To Determine If A Curve Is Smooth X And Y Intercept Graph

The concept of curvature provides a way to measure how sharply a smooth curve turns.

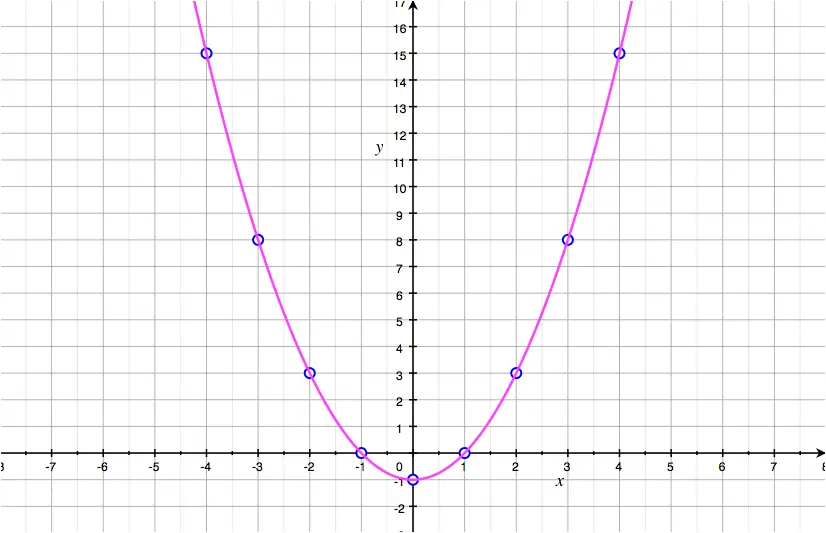
How to determine if a curve is smooth. R(t) = (2t+ 1)i + (3 t)j + tj for all t. The curve α(t) = (t3, t2) in the plane fails to be. Smooth curves are sometimes defined a little more precisely, especially in numerical analysis and complex analysis.

When we deal with curves (and f f is a curve), we say that a point a a of the domain is singular if f′(a) = 0 f ′ ( a) = 0 and regular otherwise. A curve is piecewise smooth if it has a piecewise smooth parametrization. Graphically, a smooth function of a single variable can be plotted as a single continuous.

And we say that f f is. Here r 0 (t) = 2i 1j + 1k which is The smaller the radius of the circle, the greater the curvature.

\[\mathbf{n} = \frac{d\mathbf{\hat{t}}}{ds}\mathrm{ or }. I → r3 is said to be regular if α'(t) ≠ 0 for all t ∊ i. In this video, i show that a curve described by a vector function is not smooth by showing there are values of t that make the derivative equal to zero.

It is not a smooth curve as it cannot be written as graph. In this question, for instance, a curve $\gamma \colon [a,b] \longrightarrow \mathbb{r^n}$ is defined to be smooth if all derivatives exist and are continuous.

A curve is said to be smooth if it has a tangent at each point of it and this tangent turns smoothly or continuously as the point of tangency moves along the curve. A curve \(c\) defined by \(x=f(t)\), \(y=g(t)\) is smooth on an interval \(i\) if \(f^\prime\) and \(g^{\prime}\) are continuous on \(i\) and not simultaneously 0 (except. Smooth functions have a unique defined first derivative (slope or gradient) at every point.

A curve $\mathbf{r}(t)$ is considered to be smooth if its derivative, $\mathbf{r}'(t)$, is continuous and nonzero for all values of $t$. I have to determine whether the following curves are smooth or not and i'm having trouble with the following two functions: Include expenses such as housing, utilities,.

This video explains how to determine when a curve express by a vector valued function is smooth. Recall that a parametric curve is defined. The simplest way to answer this question is to look at continuity.

A curve is smooth if every point has a. $f'(t) = (2t,2t)^{t}=$ 0 iff $t=0$. In this section we want to briefly discuss the curvature of a smooth curve (recall that for a smooth curve we require →r ′(t) r → ′ ( t) is continuous and →r ′(t) ≠ 0 r.

Equivalently, we say that α is an immersion of i into r3. Try to get a complete picture of what your monthly expenses would be in each location. In summary, normal vector of a curve is the derivative of tangent vector of a curve.